Zitat:
Zitat von BlinderBarmer
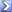
Man kennt die Varianz von TSI, und man kennt die Varianz von CO2, CO2 hat 2W/m2, TSI hat sich seit 1900 um 0,26W/m2 verändert, also gut 1/8 des Antriebs durch CO2.
|
2 besonders interessante Ansätze zur Quantifizierung des Sonneneinflusses (weil sie so generell und ziemlich zwingend sind):
Using the oceans as a calorimeter to quantify the solar radiative forcing
Errechnet aus der Amplitude des zum Sonnenzyklus korreliert schwingenen Meeresspiegels eine Amplifikation der TSI um Faktor 5 bis 7.

(Fig 2 aus dem Paper, von
hier)
Darauf beruft sich auch Svensmark (siehe Video
hier)
Wenn das stimmt, wäre der solare Einfluss etwa 3/4 (=6/8) so groß wie der CO2-Einfluss (den müsste man aber wohl durch diesen Effekt etwas nach unten korrigieren).
Solar and planetary oscillation control on climate change: hind-cast, forecast and a comparison with the CMIP5 GCMs
Approximiert die Temperaturkurve durch harmonische Schwingungen ("empirisches Modell") und kommt zu dem Ergebnis:
"
The empirical model given in Figure 16 implies that about 50-60% of the about 0.8-0.85 °C warming observed since 1850 is due to a combination of natural oscillations, including a quasimillennial cycle that was in its warming phase since 1700 AD. Since 1850 major quasi 20-year and 61-year cycles describe the GST multidecadal scales, and two decadal cycles of about 9.1 years and 10-11 years capture the decadal GST scale. Other minor oscillations of about 12, 15 and 30 year period, also linked to astronomical oscillations (see Fig. 6) appear to be present but are ignored here. The proposed six-frequency empirical climate model, Eq. 7, outperforms all CMIP3 and CMIP5 GCM runs, and predicts under the same emission scenarios a significantly lower warming for the 21st century ranging from 0.3 °C to 1.6 °C, which is an upper limit."

(Figure 16, von
hier)